
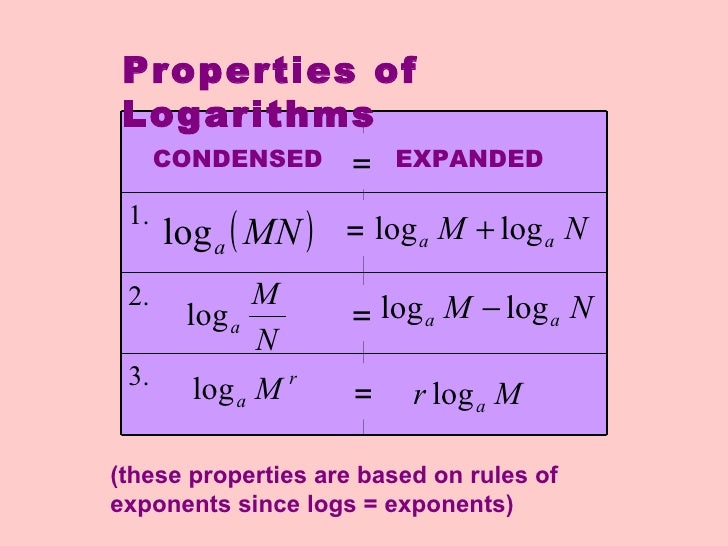
Logarithms to base e are called natural logarithms, since they occur in the life sciences and economics in natural situations that involve growth and decay. NATURAL LOGARITHMS In In most practical applications of logarithms, the number e ≈ 2.718281828 is used as base. The solution set of the given equation is _0 USING A PROPERTY OF EXPONENTS TO SOLVE AN EQUATIONįirst, write 1/3 as 3^-1, so that (1/3)^x=3^(-x).

1^4=1^5, even though 4!=5.ĮXPONENTIAL EQUATIONS The properties given above are useful in solving equations, as shown by the next examples. For Property (b) to hold, a must not equal 1 since, for example. In part (a), a!=1 because 1^x=1 for every real-number value of x, so that each value of x does not lead to a distinct real number. This means that a^x will always he positive, since a is positive. For example, (-6)^x is not a real number if x = 1/2. Properties (a) and (b) require a>0 so that a^x is always defined. (b) In a>0 and a!=1, then a^b=a^c if and only if b=c. So that when a > 1, increasing the exponent on a leads to a larger number, but if 0 0 and a!=1, then a^x is a unique real number for all real numbers x. For example, if y=2^x, then each real value of x leads to exactly one value of In addition to the rules for exponents presented earlier, several new properties are used in this chapter. With this interpretation of real exponents, all rules and theorems for exponents are valid for real-number exponents as well as rational ones. this is exactly how 2^(root(3) is defined (in a more advanced course).
Since these decimals approach the value of root(3) more and more closely, it seems reasonable that 2^(root(3) should be approximated more and more closely by the numbers to 2^(1.7),2^(1.73),2^(1.732), and so on. For example, the new symbol 2^(root(3) might be evaluated by approximating the exponent root(3) by the numbers 1.7,1.73,1.732.
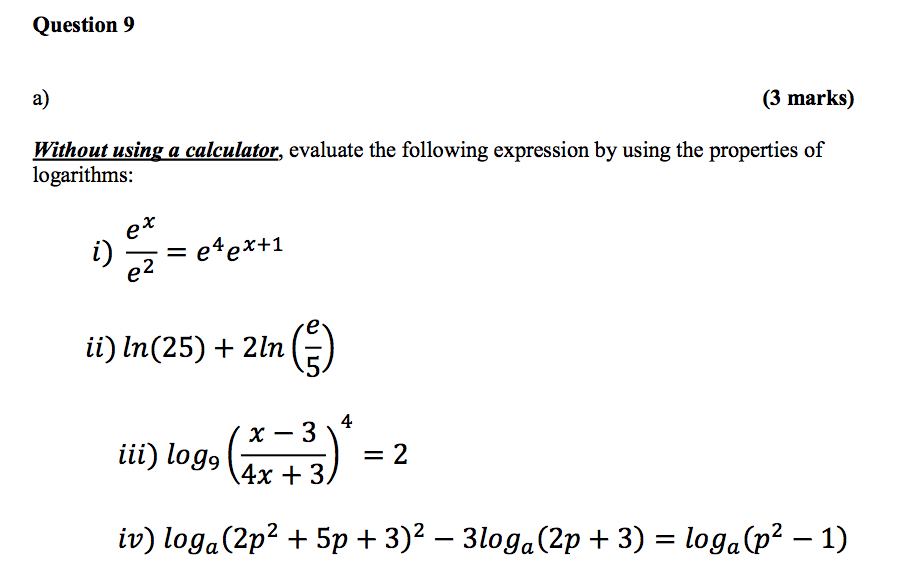
In this section the definition of a^r is extended to include all real (not just rational) values of the exponent r.


 0 kommentar(er)
0 kommentar(er)
